Simulation of collective dynamics oF microscale swimmers
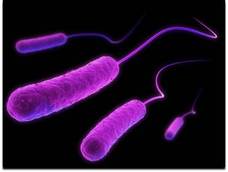E Coli in motion (Source: Wikepedia)
Recent experiments have shown that suspensions of swimming micro-organisms are characterized by complex dynamics involving enhanced swimming speeds, large-scale correlated motions and enhanced tracer diffusion. In this work we develop a particle-based computational model to study a suspension of hydrodynamically interacting rod-like swimmers with the relation between the swimming velocity and intrinsic stress being enforced from slender body theory. Such an a priori specification reduces the computational cost since one now has a ‘kinematic’ simulation with a fixed interaction law between swimmers; this does not restrict our study of the dynamics since the destabilizing mechanism has been attributed to the intrinsic (rather than the induced) stress field. Importantly, the model will include intrinsic de-correlation mechanisms found in bacteria such as rotary diffusion and tumbling whose effects have so far not been studied via simulations. We show that a suspension of smooth swimmers (devoid of de-correlation mechanisms) has a box size dependent stability threshold. We are currently using this model to explore a box-size independent stability threshold based on the suspension concentration, tumble-time (duration between subsequent tumble events) and rotary diffusivity as predicted by Subramanian & Koch (JFM 2009).
